

Answer: D
Solution:
The three prices round to $2, $5, and $10, which has a sum of 17.
中文解析:
三件商品价格先近似取整,然后求和:2+5+10=17. 答案是D。

Answer: C
Solution:
As the AMC 8 only rewards 1 point for each correct answer, everything is irrelevant except the number Billy answered correctly,13.
中文解析:
正确的题目每题1分, 错误或没做的题目都是0分,做对13题的得分应该是13. 答案是C。
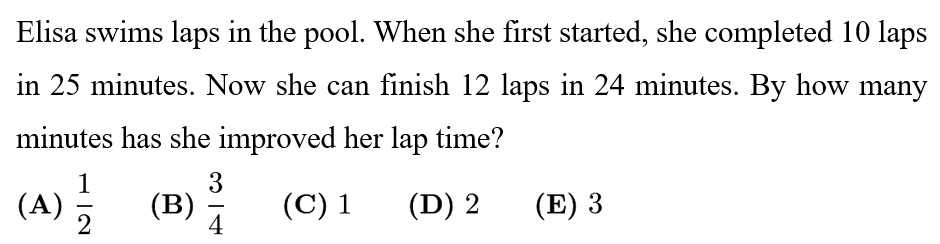
Answer: A
Solution:
When Elisa started, she finished a lap in 25/10=2.5 minutes. Now, she finishes a lap is 24/12=2 minutes. The difference is 2.5-2=0.5
中文解析:
开始25分钟游10圈,平均2.5分钟游1圈。 后来24分钟游12圈,平均2分钟游1圈。 速度从2.5分钟提高到2分钟,提高了0.5分钟,即1/2 分钟。 答案是A。
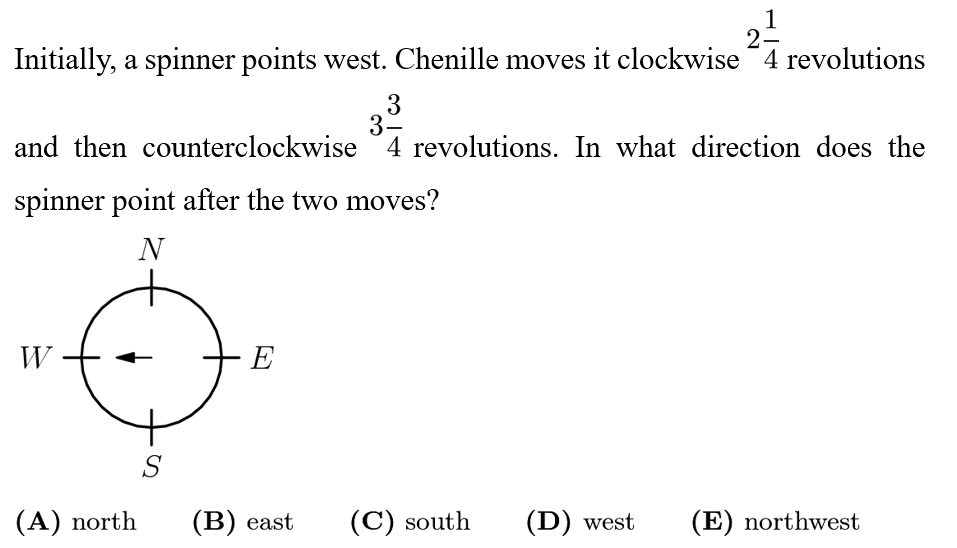
Answer: B
Solution:
If the spinner goes clockwise 2+1/4 revolutions and then counterclockwise 3+3/4 revolutions, it ultimately goes counterclockwise 1+1/2 which brings the spinner pointing east.
中文解析:
最初方向指向西, 转整数圈不改变指针方向。 顺时针转1/4圈之后指向北。逆时针转3/4圈后指向东。 答案是B。

Answer: D
Solution:
Drawing segments AC and BD, the number of triangles outside square ABCD is the same as the number of triangles inside the square. Thus areas must be equal so the area of ABCD is half the area of the larger square which is 60/2=30.

中文解析:
链接AC和DB,相交于点O。在小正方形AEDO中,AED和AOD相等。 即ADO 是AEDO的一半。 由于图形的对称性,可知ADCB是大正方形EFGH的一半,所以面积是30. 答案是D。

Answer: C
Solution:
The perimeter of two rectangles would be 24. The final perimeter is 24-4=20.
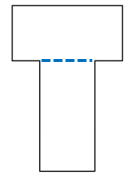
中文解析:
1个长方形的周长是12. 这两个长方形拼起来周长是24. 但有两条长度为2的边重合,因此周长是24-4. 即20. 答案是C。

Answer: B
Solution:
Using the formulas of circles, C=2*Pi*r and A=Pi*r*r, we find that circle Y has a radius of 4 and circle Z has a radius of 3. Thus, the order from smallest to largest radius is Z, X, Y.
中文解析:
圆X的半径是Pi,即3.14; 圆Y的周长是8Pi,所以半径是4; 圆Z的面积是9Pi,所以半径是3. 半径从小到大的顺序是Z, X, Y。 答案是B。
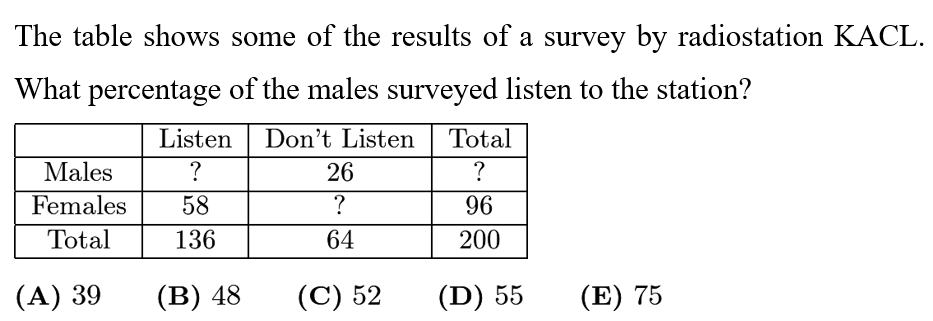
Answer: E
Solution:
Males which listen are (136-58)=78. The total males which surveyed are 78+26=104. Thus the percentage of males surveyed listen to the station is 78/104=75%.
中文解析:
收听的男性人数是: 136-58=78人。 不听的男性是26人,合计男性: 78+26=104人。 收听的比例是: 78/104. 大约是75%。 答案是E。
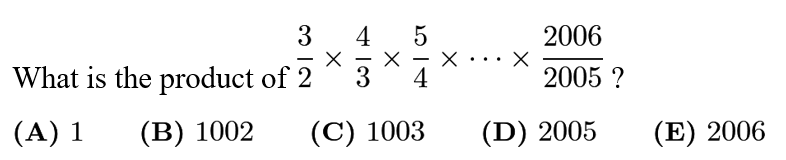
Answer: C
Solution:
After looking at the problem, we immediately notice that terms cancel out, leaving us with 2006/2=1003.
中文解析:
分子分母约分(simplify)之后剩下:2006/2,即1003. 答案是C。
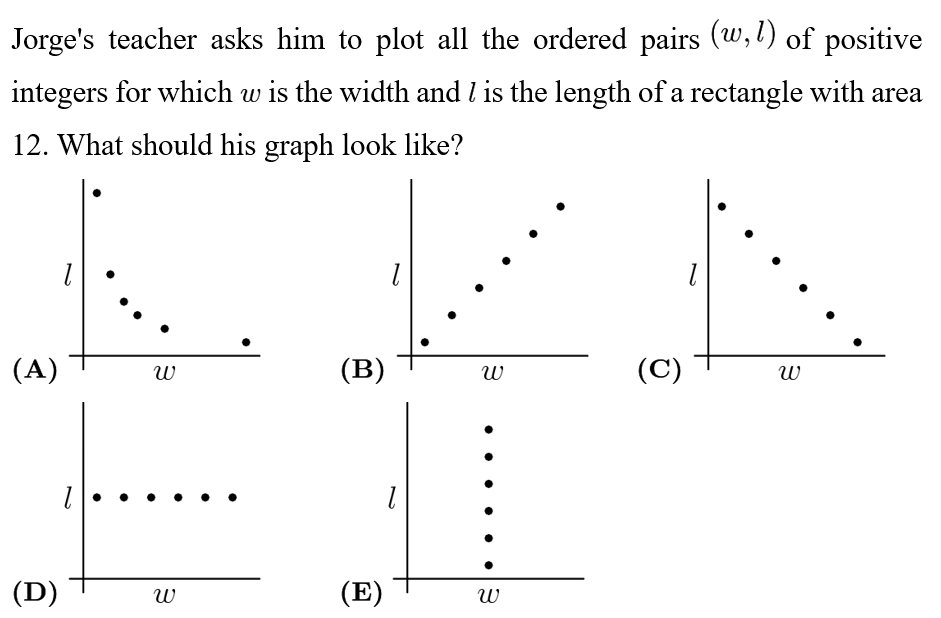
Answer: A
Solution:
The length of the rectangle will relate invertly to the width, specifically using the theorem l=12/3. The only graph that could represent a inverted relationship is A. (The rest are linear graphs that represent direct relationships, therefore they are incorrect.)
中文解析:
长方形的面积是12, 我们让宽逐渐增大(宽和长都是正整数),当w=1时,l=12; 当w=2时, l=6; 当w=3时, l=4; 当w=4时, l=3; 当w=6时, l=2; 当w=12时, l=1; 可以看出,随着w的增大, l 逐渐变小,只有选项A, 选项C符合这个趋势。 此外l和w的变化不是线性变化,排除C, 答案是A。
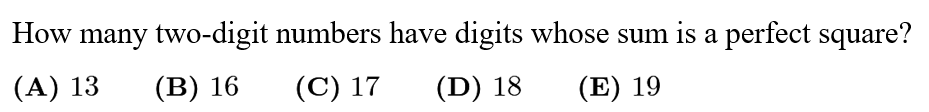
Answer: C
Solution:
There is 1 integer whose digits sum to 1,即10.;There are 4 integers whose digits sum to 4: 13,22, 31, 40. ; There are 9 integers whose digits sum to 9: 18, 27, 36, 45, 54, 63, 72, 81, 90. ; There are 3 integers whose digits sum to 16: 79, 88, 97. Two digits cannot sum to 25 or any greater square since the greatest sum of digits of a two-digit number is 9+9=18. Thus, the answer is 1+4+9+3=17.
中文解析:
两个数字的和最大是9+9=18, 而18以内的平分数只有1,4,9,16. 个位数与十位数的和是1的两位数只有“10”。 个位数与十位数的和是4的两位数有“13”,“22”,“31”,“40”。个位数与十位数的和是9的两位数有“18”,“27”,“36”,“45”,“54”,63“,”72“,”81“,”90“。 个位数与十位数的和是16的两位数有“79”,”88“,”97“。 这些两位数合计有1+4+9+3=17个。 答案是C。

Answer: D
Solution:
70%*10=7; 80%*20=16; 90%*30=27. Adding them us gets 7+16+27=50. The overall percentage correct would be 50/60=83.
中文解析:
10个题目中做对了7个; 20个题目中做对了16个;30个题目中做对了27个。 共计做对了50个。 即60个题目中做对了50个,正确率是83%。 答案是D。

Answer: D
Solution:
If Cassie leaves half an hour earlier then Brian, when Brian starts, the distance between them will be 62-12/2=56. Every hour, they will get 12+16=28 miles closer. 56/28=2, so 2 hours from 9:00 AM is when they meet, which is 11:00.
中文解析:
两人的距离是62英里, 9:00时,Cassie已经行驶的距离是0.5*12=6英里。 也就是说,9点钟的时候Cassie和Brian之间的距离是62-6=56英里。 我们可以理解为两个人在9点钟的时候,相距56英里,同时出发。 一个速度是12,一个速度是16,相向而行,合计速度是28. 那么时间是:56/28=2小时。 即11点相遇。


Answer: B
Solution:
760*45-760*30=760*15=11400.
中文解析:
Bob需要的时间是760*45秒, Chandra需要的时间是760*30秒。 Bob多用了760*45-760*30,即11400秒。 答案是B。

Answer: C
Solution:
we set up an equation and solve. Let x be the number of pages that Chandra reads. 30*x=45*(760-x), thus x=456.
中文解析:
假设C读x页,则B读(760-x)页。 C读书花的时间是30x秒,B读书花的时间是45*(760-x)秒,B和C读书所花费的时间相同,即得到等式:30*x=(760-x)*45. 从而x=456. 答案是C。

Answer: E
Solution:
The amount of pages Bob, Chandra, and Alice will read is in the ratio 4:6:9. Therefore, Bob, Chandra, and Alice read 160, 240, and 360 pages respectively. They would also be reading for the same amount of time because the ratio of the pages read was based on the time it takes each of them to read a page. Therefore, the amount of seconds each person reads is simply 160*45=7200.
中文解析:
三个人读书的时间相同,假设都是t秒,则A读的页数是t/20; B读的页数是t/45;C读的页数是t/30; 三个人合起来把这本书读完了,因此: t/20+t/45+t/30=760. 从而t=7200. 答案是E。
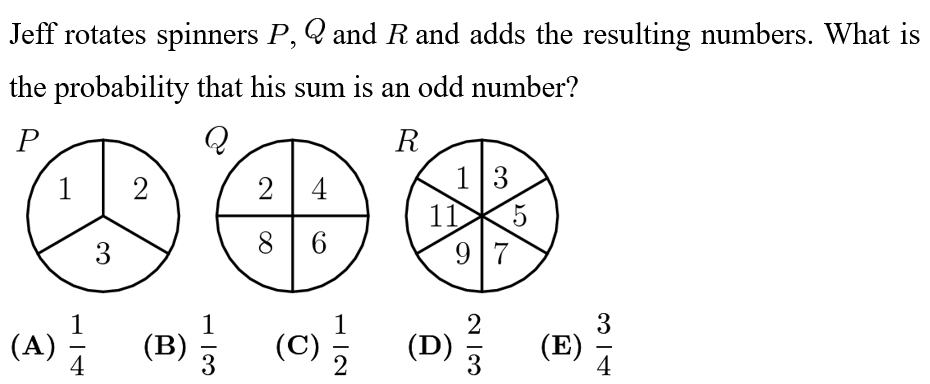
Answer: B
Solution:
In order for Jeff to have an odd number sum, the numbers must either be Odd + Odd + Odd or Even + Even + Odd. We easily notice that we cannot obtain Odd + Odd + Odd because spinner Q contains only even numbers. Therefore we must work with Even + Even + Odd and spinner Q will give us one of our even numbers. We also see that spinner R only contains odd, so spinner R must give us our odd number. We still need one even number from spinner P. There is only 1 even number: 2. Since spinning the required numbers are automatic on the other spinners, we only have to find the probability of spinning a 2 in spinner P, which is clearly 1/3.
中文解析:
转盘Q都是双数,转盘R都是单数, 如果希望三个转盘的结果数字相加的和是单数,则P必须是双数。 P上有3个数,只有1个数是双数,因此P的结果是双数的概率是1/3. 即是本题所求的概率。 答案是B。
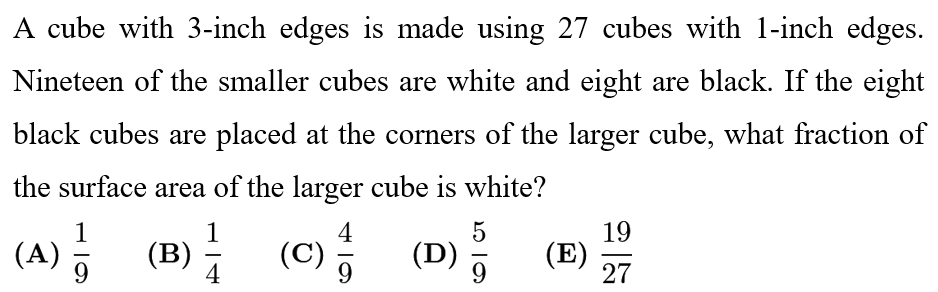
Answer: D
Solution:
The surface area of the cube is 6*3*3=54. Each of the eight black cubes has 3 faces on the outside, making 3*8=24 black faces. Therefore there are 54-24=30 white faces. To find the ratio, we evaluate 30/54=5/9.
中文解析:
在corner处的小正方体,每个小正方体暴露出来3个面,一个大正方体共有8个corner。 所以大正方体的表面积中黑色的面积是8*3=24. 大正方的表面积是: 3*3*6=54. 因此白色的占比是(54-24)/54=5/9. 答案是D .
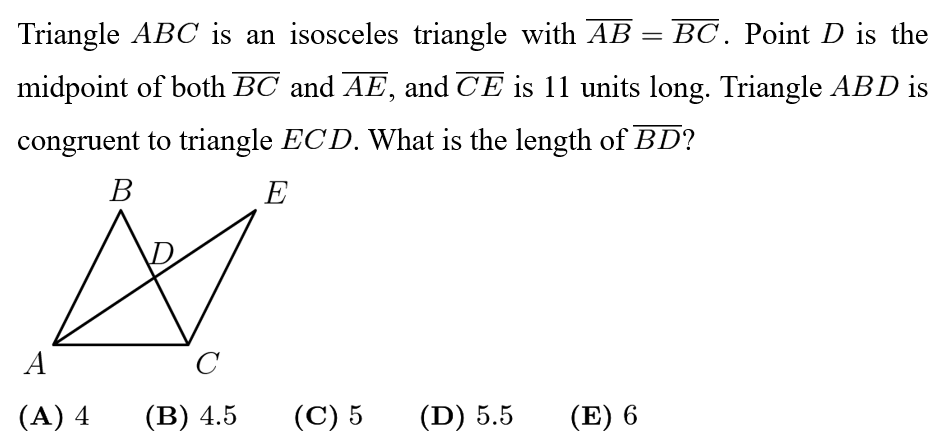
Answer: D
Solution:
Since triangle ABD is congruent to triangle ECD and CE=11, AB=11. Since AB=BC, BC=11. Because point D is the midpoint of BC, BD=11/2=5.5.
中文解析:
因为三角形ABD和三角形ECD全等,所以AB=EC=11. 三角形ABC是等腰三角形,AB=BC ,所以BC=11. D是BC的中点,所以BD=11/2=5.5. 答案是D。

Answer: C
Solution:
Since there are 6 players, a total of 6*5/2=15 games are played. So far, 4+3+2+2+2=13 games finished (one person won from each game), so Monica needs to win 15-13=2.
中文解析:
6个队伍中,每个队都要和其他队比赛一次,这是一个从6个队伍中,任选出2个队伍进行一场比赛的组合问题,即C(6,2)。 根据组合公式求得15. 一直比赛场次4+3+2+2+2=13, 因此还需要比赛2场,即Monica需要赢2场。 答案是C。

Answer: A
Solution:
The water level will rise 1cm for every 100*40=4,000 . Since 1,000 is 1/4 of 4,000, the water will rise 1/4=0.25.
中文解析:
如果高是1cm, 对应的体积是100*40*1,即4000. 体积为1000的石块,只能因此因为增加1000/4000,即1/4 cm. 答案是A。
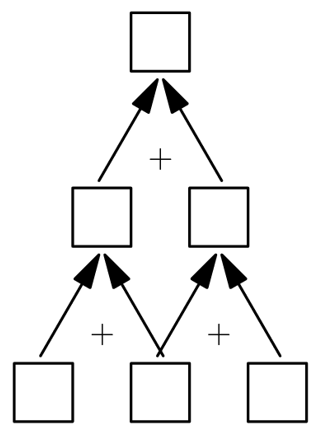
Answer: D
Solution:
If the lower cells contain A,B, and C, then the second row will contain A+B and B+C , and the top cell will contain A+2B+C. To obtain the smallest sum, place 1 in the center cell and 2 and 3 in the outer ones. The top number will be 7. For the largest sum, place 9 in the center cell and 7 and 8 in the outer ones. This top number will be 33. The difference is 33-7=26.
中文解析:
若想最高的那行取得最大值,最底部的那行必须是最大的个位数。 由于最底层中间的位置在计算第二行的数据时被用了两次,因此让最底行的中间的那个数取最大值即9. 最底行的另两个数是8和7. 这样第二行的数据就分别是17和16, 最高行是33. 同样道理,若想最高行取得最小值,底层中间是1,另两个是2和3, 中间行是3和4, 最高行是7. 最大值与最小值的差就是33-7=26. 答案是D。

Answer: A
Solution:
x=6*q1+4; => x+2=6*(q1+1);
x=5*q2+3; =>x+2=5*(q2+1);
If there were two more coins in the box, the number of coins would be divisible by both 6 and 5. The smallest number that is divisible by 6 and 5 is 30, so the smallest possible number of coins in the box is 28 and the remainder when divided by 7 is 0.
中文解析:
金币的个数除以6,余数是4, 除以5,余数是3. 我们先计算6 和5 的最小公倍数,是30. 如果金币数量增加2个,则除以6时就能被整除,除以5时也能被整除,因此金币个数是30-2=28个。 28个金币分给7个人时,余数是0. 答案是A。

Answer: A
Solution:
CD*101=CDCD. So ABA=101, A=1,b=0. A+B=1.
中文解析:
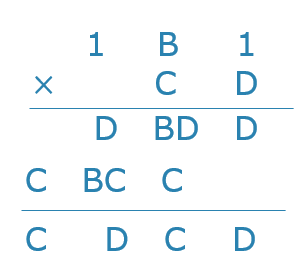
个位数: A*D=D,推测A=1。 把A带入, 变成1B1*CD, 按照乘法规律列竖式, 如上图所示。 十位数 BD+C=C , 百位数D+BC=D, 推出B=0. 因此A+B=1. 答案是A。
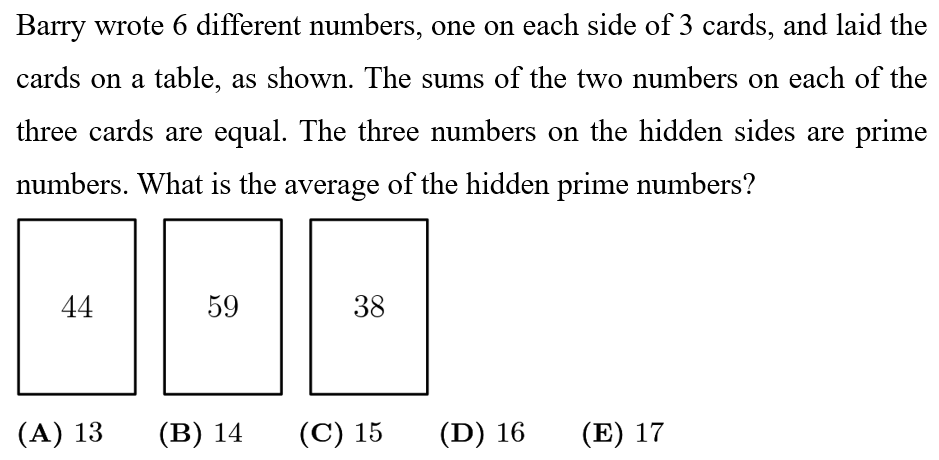
Answer: B
Solution:
Notice that 44 and 38 are both even, while 59 is odd. If any odd prime is added to 59, an even number will be obtained. However, the only way to obtain this even number would be to add another even number to 44, and a different one to 38. Since there is only one even prime (2), the middle card's hidden number cannot be an odd prime, and so must be even. Therefore, the middle card's hidden number must be 2, so the constant sum is 59+2=61. Thus, the first card's hidden number is 61-44=17, and the last card's hidden number is 61-38=23. Since the sum of the hidden primes is 2+17+23=42. the average of the primes is 42/3=14.
中文解析:
三张牌的隐藏的面的数字都是质数,三张牌显露出来的数字分别是44,59,38, 这三个数字有单数,有双数, 如果三个不同的质数和44,59,38的和相同,则其中一个质数必然是2. 因为质数里唯一的双数是2. 2+59=61。 因此另一个质数是61-44=17, 另一个是61-38=23。 这三个质数分别是2, 17,23, 其平均数是14. 答案是B。
AMC培训、答疑,请联系微信 / 电话:136 1118 1627
艾蕾特教育 @Elite Edu, 在这里,